It may be possible in practice that the alternator running with full
excitation may undergo a sudden short circuit because of the abnormal
conditions. Due to sudden short circuit of alternator, large mechanical
forces are developed which may not be sustained by the alternator. These
forces are proportional to square of the current value, hence large
pressure is built up between adjacent stator conductors.
 |
| Fig. 1 Construction for deriving capability curve of synchronous generator |
The short circuit transients in a synchronous machine is a
complicated phenomena due to number of circuits coupled to each other
are involved. When a synchronous generator undergoes short circuit, it
has a characteristics time varying behaviour. During short circuit, flux
per pole dynamically changes. Thus the transients are seen in the field
and damper windings. The alternator can be represented by an equivalent
circuit wherein the reactance is seen to be changed from subtransient
reactance to final steady state synchronous reactance.
When alternator undergoes a short circuit number of events take
place which depends on various factors such as the instant in the cycle
at which short circuit occurs, whether the machine is loaded or not,
what is the excitation provided, how many phases are involved, whether
it is occuring near to machine terminals or away from it and on the
constructional features of the machine. Hence the evaluation of sudden
short circuit current for the given conditions is complex and to some
extent empirical process depending on values of resistance, self and
mutual inductances which themselves are variable and difficult to
assess.
After the moment of short circuit, the time period followed by it
can be divided into three periods. The first one is very short period
of one or two cycles the conditions of which are dependent on the flux
linkages between stator and rotor during short circuit. The second
interval is longer one which is nothing but transient decay of short
circuit current which is affected by damping and rise of armature
reaction. The final period is nothing but the steady state short circuit
before which the generator is normally open circuited.
1.1 Constant Flux Linkage Theorem
The behaviour shown by the alternator just after short circuit
can be understood by the use of constant linkages theorem. If a closed
circuit with resistance r and inductance L is considered without a
source then the equation obtained using KVL will be ri + L (di/dt) = 0.
If r is very very small then L(di/dt) = 0 or d/dt (LI) = 0 This shows
that the flux linkages Li remain constant. In generator also the
effective inductance of stator and rotor windings is large compared to
the resistance which can be neglected for first few cycles. The rotor
circuit is closed through exciter while stator is closed by short
circuit. Thus the flux linking with either winding must remain constant
irrespective of the rotation.
1.2 Analysis of RL Series Circuit
Similar to theorem of constant linkages let us consider a series
R-L circuit excited by a voltage source which is sinusoidal for further
understanding of short circuit.
The circuit diagram is as shown in the Fig. 2.Let at the instant t = t1, the sinusoidal voltage V sint is applied to series R-L circuit.
Applying KVL,
Ri + L(di/dt) = V sinω t ................. (1)
For the above equation the complementary function of the solution is
iCF = K1 e-(R/L)t
For obtaining the particular solution let the trial solution be
iPI = A cosωt + Bsinωt
where A and B are undetermined coefficients.
i'PI = - A sin ωt(ω) + B cosωt(ω)
i'PI = -A ωsinωt + Bω cosωt
Substituting trial solution and its derivative in equation (i)
R(A cosωt + B sinω t) + L ( -A sinωt + Bωcosω t) = V sin t
(RB - LAω) sinωt + (RA + ωLB) cosωt = Vsinωt
Comparing coefficient of like terms
RB - LAω = V
RA + ωLB = 0
Solving above equations we get
The particular solution is therefore given by,
The complete solution for i is given by,
To find the value of K1 let us use initial conditions i.e.
Hence the complete solution is given as,
Substituting in above equation,
The corresponding waveforms are as shown in the Fig. 3.
 |
| Fig. 3 |
The first term in the above equation represents steady state current (is) while the second term represents transient current (it). If the voltage is switched at t = t1 when it is zero, the transient term is having the greatest value. The resultant current is zero having complete asymmetry. The approximate current in this case reaches 2im which is known as doubling effect compared to the switching of voltage at the instant when voltage is at its maximum instead of zero. This shows that the current flowing in the circuit changes its waveform if the instant at which voltage is applied to the circuit, is changed. The same thing is applicable in case of generator undergoing short circuit.






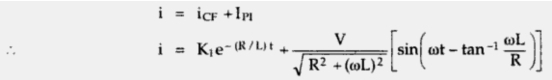






No comments:
Post a Comment